Resistors and AC Voltage, Current & Power

Pure resistive AC circuit: resistor voltage and current are in phase.

If we were to plot the current and voltage for a very simple AC circuit consisting of a source and a resistor, it would look something like this:

Voltage and current “in phase” for resistive circuit.

Because the resistor follows Ohm’s law and simply and directly resists the flow of electrons at all periods of time, the waveform for the voltage drop across the resistor is exactly in phase with the waveform for the current through it. In other words, for every point in time, [latex]e=iR[/latex].

We can look at any point in time along the horizontal axis of the plot and compare the values of current and voltage with each other. When the instantaneous value (any “snapshot” look at the values of a wave are referred to as instantaneous values) for current is zero, the instantaneous voltage across the resistor is also zero. Likewise, at the moment in time where the current through the resistor is at its positive peak, the voltage across the resistor is also at its positive peak, and so on. At any given point in time along the waves, Ohm’s Law holds true for the instantaneous values of voltage and current.

We can also calculate the power dissipated by this resistor ([latex]p=ie[/latex]), and plot those values on the same graph:

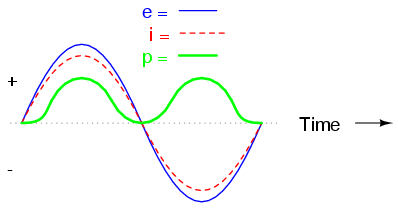
Instantaneous AC power in a pure resistive circuit is always positive.

Note that the power is never a negative value. When the current is positive (above the line), the voltage is also positive, resulting in a power ([latex]p=ie[/latex]) of a positive value. Conversely, when the current is negative (below the line), the voltage is also negative, which results in a positive value for power (a negative number multiplied by a negative number equals a positive number). This consistent “polarity” of power tells us that the resistor is always dissipating power, taking it from the source and releasing it in the form of heat energy. Whether the current is positive or negative, a resistor still dissipates energy.

Ohm’s Law Calculations in AC

Ohm’s law still applies to resistors in AC circuits: [latex]E=IR[/latex]

Or, more correctly: [latex]e=iR[/latex] because we use lower case [latex]e[/latex] and [latex]i[/latex] (and [latex]p[/latex] ) to denote that the voltage/current/power is AC instead of DC.

However, as we learned in chapter 1, the voltage or current of an AC signal can be represented in multiple different ways. You can use the peak voltage (current), the peak-to-peak voltage (current), or RMS voltage (current) to describe it. So which one should you use to calculate Ohm’s Law? Well it turns out that it doesn’t matter, as long as you’re consistent.

For example (assume all voltages and currents are sinusoidal):

• If you have a 100V peak voltage applied across a 10 Ω resistor, you would have a [latex]\frac{100V}{10\Omega} = 10A[/latex] peak current
• if you have a 10mA peak-to-peak current going through a 1kΩ resistor, you would have a [latex]0.1A\times1000\Omega=10V[/latex] peak-to-peak current across it
• If you have apply 120V_rms to a resistor and get 1A peak current, the resistor would not be [latex]\frac{120V}{1A}=120\Omega[/latex] because the voltage and current are two different types of amplitudes, you would need to convert the peak current to RMS ([latex]\frac{1A}{\sqrt{2}} = 0.707A[/latex]) to get [latex]\frac{120V}{0.707A}=169.7\Omega[/latex]

Since voltage across and current through a resistor are in phase with each other, there is no need to include the phase when doing analysis or calculations on AC circuits that only have resistors. However, when we start to include inductors and capacitors, we will have to take into account phase, so as a simple introduction, using phase in AC circuit analysis, let’s look at how to do that with resistors.

Vector Representation of Resistors: Impedance

When plotting out power for a resistor earlier, we saw that the power is always positive – all of the energy put into a resistor when current flows through it is dissipated as heat. When the power is dissipated like this, it is considered real power.

As we will see with inductors and capacitors, current flow only results in a flow of electrical energy into and out of the device, energy is not dissipated as heat. We’ll talk more about this in the next chapters, but for now, understand that this type of power transfer is considered imaginary because it is not used up.

Now, think back to chapter 2 – having a real part and an imaginary part sounds a lot like the complex plane. Resistors only have a real part, but they can still be represented by a complex number with the imaginary part set to zero. For example a 10 Ω resistor in an AC circuit can be represented as [latex]10+j\Omega[/latex] or [latex]10\Omega\angle0^o[/latex]

For resistors (and inductors and capacitors), this complex number is called the impedance of the device and is represented by the letter Z (in bold to indicate that it is a vector).

(Side note: multiple devices can be combined together to create a total impedance for a circuit).

Another way to think about the affect of resistors (and inductors and capacitors) on voltage and current in AC circuits, is to consider the two different ways that the components affect the relationship between voltage and current. It is not obvious at this point, but not only do these components affect the relationship of magnitude between voltage and current, but they also affect the phase difference between voltage and current (resistors “cause” no phase shift, but inductors and capacitors do).

To represent the two different effects, we can use a vector (the impedance) to represent the magnitude of the effect and the direction of the effect. For resistors, the magnitude of the effect is the resistance (which as we know from Ohm’s law is the ratio of voltage across and current through the resistor) and the direction of the effect is 0 since resistors cause no phase shift.

Examples

[h5p id=”3″]

Resistors in Series and Parallel

Impedances can be combined. When components are in series and parallel, the overall impedance is calculated in the same way as for DC circuits except that all of the arithmetic is vector arithmetic. Resistors are a simple case though since they have no imaginary component. If you have only resistors in an AC circuit, you can combine them to get an overall equivalent impedance in exactly the same way you would in a DC circuit.

Examples

[h5p id=”4″]

[h5p id=”5″]

Contributors

Contributors to this chapter are listed in chronological order of their contributions, from most recent to first.

David Williams (2022): Separated resistors into its own chapter. Added videos, added sections on vector representation of resistors

CC-BY 2000-2020 Tony R. Kuphaldt.